ACCA PM Chapter 7 - PRICING - Optimal pricing – equations

Price\Demad Equation

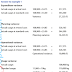
P = a - bQ
where,
P = Selling Price
Q= Quantity Demanded at that Price
a= Theoretical Maximum Price. (The demand will be zero)
b= the change in price required to change demand by 1 unit (Gradient)

Optimum Selling Price

Steps:

1. Establish the demand function; (find a and b); b = change in price/change in quantity
2. Establish the marginal cost; Fixed overheads are ignored as they are not part of the marginal cost.
3. Establish the marginal revenue function: MR = a - 2bQ; using MC = MR.
4. Solve the MR function the determine the optimum quantity, Q.
5. Insert the value of Q from Step 4 into the demand function determined in step 1 and calculate the optimum price.
6. Calculate profit; (Revenue - Variable costs - Fixed costs = Profit).

Source: https://opentuition.com/acca/pm/acca-performance-management-pm-lectures/